Sports categories and titles: list and assignment

Receiving one or another rank is a serious step from amateur to professional sports. And the conferment of the title is already well-deserved recognition of the achievements of the eminent athlete. But many are confused about existing Russian sports ranks and ranks, their order. We will try to clarify with this article.
Sports titles and categories
Athletes are assigned ranks at the beginning of their careers, and upon reaching all the latter, ranks. The ascent to the podium begins with youth sports categories:
- 3rd youth;
- 2nd youth;
- 1st youth;
- 4th category (applicable only in chess - you need to play at least 10 games and score at least 50% of the points in a group game);
- 3rd category;
- 2nd category;
- 1st category.
Let us note that youth ranks are awarded only in those types of sports where age is a decisive factor in competitions, where strength, endurance, reaction speed, and speed of the participant are important. Where it is not an important advantage or disadvantage (for example, in intellectual sports), the youth rank is not assigned.
Those who have the 1st sports category can already be awarded titles. We list them in ascending order:
- master of Sport;
- international master of sports/grandmaster;
- deserved
A long-standing custom prescribes that international-level masters of sports should be named intellectual games(checkers, chess, etc.) by grandmasters.
About EVSK
In the Russian Federation, confirmation and assignment of sports categories and titles is determined by a document called the Unified All-Russian Sports Classification (EVSK). It indicates the standards in each sport that must be fulfilled in order to receive a certain category and title. The first such document was approved in 1994; accepted by Evsk for four years. Today the option is valid for 2015-2018, for summer - 2014-2017.

The document is based on the All-Russian Register of Sports and the list of sports recognized by the Ministry of Sports of the Russian Federation sports games. The document dictates both the standards that must be met to obtain a particular sports category or title, and the conditions under which all this must occur: the level of the opponent, the importance of the competition, the qualifications of the judges.
Why do you need a sports category?
The assignment of ranks in sports has several clearly defined goals:
- Mass popularization of sports.
- An incentive to improve the level of sports training and skill.
- Moral encouragement of athletes.
- Unification of assessments of achievements and mastery.
- Approval of a uniform procedure for assigning sports categories and titles for all.
- Development and continuous improvement of the field physical culture and sports.
Assignment procedure
Let's touch on the general important points assignment of ranks and categories:

- Athletes must be divided into juniors, young people, and adults.
- A young athlete who took part in a scheduled competition and fulfilled the necessary standards for a certain category receives the latter. This will be evidenced by a badge and a special qualification book.
- The athlete’s record book must be registered with the organization where he received this document. In the future, at all competitions in which the athlete will participate, he will enter into this qualification book all the information about his results in competitions, assigned and confirmed categories, and prizes won. Each entry is made based on a specific protocol and is certified by a signature responsible person and the seal of the sports organization that organized the competition.
- The assignment of a sports title is the prerogative of the Ministry of Sports of the Russian Federation. To confirm this, the athlete receives a certificate and an honorary
Requirements for the assignment of ranks and titles
Now let’s look at the requirements that an athlete must fulfill, and what he must meet in order to receive a certain rank:

- The basis for assigning a rank is only a certain measurable result of sports activity: taking a specific place in official games or competitions, achieving last year a certain number of victories over opponents of a specific level, fulfillment of a number of quantitative standards in sports where they are possible.
- Each category or title implies that the athlete has reached a certain age.
- If, within the framework of a competition, athletes are assigned categories and titles, then it must comply with a whole set of strict rules: composition and level of participants, a certain number of judges and athletes, number of performances, fights and games in the qualifying and main stages.
- At international competitions it is additionally determined smallest number participating countries. To receive the title of international master of sports or grandmaster, you must participate in competitions of this level.
- Higher ranks are awarded only to citizens of the Russian Federation and only by the Federal Agency for Physical Education and Sports.
- The categories are authorized to assign regional executive bodies spheres of physical education and sports.
- An athlete must confirm his sports category at least once every two years.
All categories and titles of sports in the Russian Federation are regulated by the EVSK. After receiving one or another category in the given order and within the framework of current requirements, the athlete must also periodically confirm it.
The digits in multi-digit numbers are divided from right to left into groups of three digits each. These groups are called classes. In each class, the numbers from right to left indicate the units, tens and hundreds of that class:
The first class on the right is called class of units, second - thousand, third - millions, fourth - billions, fifth - trillion, sixth - quadrillion, seventh - quintillions, eighth - sextillion.
For ease of reading the recording multi-digit number, a small gap is left between classes. For example, to read the number 148951784296, we highlight the classes in it:
and read the number of units of each class from left to right:
148 billion 951 million 784 thousand 296.
When reading a class of units, the word units is usually not added at the end.
Each digit in the notation of a multi-digit number occupies a certain place - position. The place (position) in the record of a number on which the digit stands is called discharge.
The counting of digits goes from right to left. That is, the first digit on the right in a number is called the first digit, the second digit on the right is the second digit, etc. For example, in the first class of the number 148,951,784,296, digit 6 is the first digit, 9 is the second digit, 2 - third digit:

Units, tens, hundreds, thousands, etc. are also called bit units:
units are called units of the 1st category (or simple units)
tens are called units of the 2nd digit
hundreds are called 3rd digit units, etc.
All units except simple units are called constituent units. So, ten, hundred, thousand, etc. are composite units. Every 10 units of any rank constitutes one unit of the next (higher) rank. For example, a hundred contains 10 tens, a ten contains 10 prime units.
Any compound unit compared to another unit smaller than it is called unit of the highest category, and in comparison with a unit greater than it is called unit of the lowest category. For example, a hundred is a higher-order unit relative to ten and a lower-order unit relative to a thousand.
To find out how many units of any digit there are in a number, you need to discard all the digits representing the units of lower digits and read the number expressed by the remaining digits.
For example, you need to find out how many hundreds there are in the number 6284, i.e. how many hundreds are in the thousands and hundreds of a given number together.
In the number 6284, the number 2 is in third place in the units class, which means there are two prime hundreds in the number. The next number to the left is 6, meaning thousands. Since every thousand contains 10 hundreds, 6 thousand contain 60 of them. In total, therefore, this number contains 62 hundreds.
The number 0 in any digit means the absence of units in this category. For example, the number 0 in the tens place means the absence of tens, in the hundreds place - the absence of hundreds, etc. In the place where there is a 0, nothing is said when reading the number:
172 526 - one hundred seventy two thousand five hundred twenty six.
102 026 - one hundred two thousand twenty six.
Our first lesson was called numbers. We have covered only a small part of this topic. In fact, the topic of numbers is quite extensive. It has a lot of subtleties and nuances, a lot of tricks and interesting features.
Today we will continue the topic of numbers, but again we will not consider it all, so as not to complicate learning unnecessary information, which at first is not really needed. We'll talk about discharges.
Lesson contentWhat is a discharge?
If we talk in simple language, then the digit is the position of the digit in the number or the place where the digit is located. Let's take the number 635 as an example. This number consists of three digits: 6, 3 and 5.
The position where the number 5 is located is called units digit
The position where the number 3 is located is called tens place
The position where the number 6 is located is called hundreds place
Each of us has heard such things as “units”, “tens”, “hundreds” since school. The digits, in addition to playing the role of the position of the digit in the number, tell us some information about the number itself. In particular, the digits tell us the weight of the number. They tell you how many units, how many tens, and how many hundreds there are in a number.
Let's return to our number 635. In the units place there is a five. What does this mean? And this means that the ones digit contains five ones. It looks like this:

In the tens place there is a three. This tells us that the tens place contains three tens. It looks like this:

There is a six in the hundreds place. This means that there are six hundreds in the hundreds place. It looks like this:

If we add up the number of resulting units, the number of tens and the number of hundreds, we get our original number 635
There are also higher digits such as the thousand digit, the tens of thousands digit, the hundreds of thousands digit, the millions digit and so on. We will rarely consider such large numbers, but nevertheless it is also desirable to know about them.
For example, in the number 1,645,832, the units place contains 2 units, the tens place - 3 tens, the hundreds place - 8 hundreds, the thousands place - 5 thousand, the tens of thousands place - 4 tens of thousands, the hundred thousand place - 6 hundred thousand, the millions place - 1 million.

At the first stages of studying digits, it is advisable to understand how many units, tens, hundreds a particular number contains. For example, the number 9 contains 9 ones. The number 12 contains two ones and one ten. The number 123 contains three ones, two tens and one hundred.
Grouping items
After counting some items, ranks can be used to group these items. For example, if we count 35 bricks in the yard, then we can use discharges to group these bricks. In the case of grouping objects, the ranks can be read from left to right. Thus, the number 3 in the number 35 will indicate that the number 35 contains three tens. This means that 35 bricks can be grouped three times in ten pieces.
So, let’s group the bricks three times ten pieces each:

It turned out to be thirty bricks. But there are still five units of bricks left. We will call them as "five units"

The result was three dozen and five units of bricks.
And if we did not group the bricks into tens and ones, then we could say that the number 35 contains thirty-five units. This grouping would also be acceptable:

The same can be said about other numbers. For example, about the number 123. Earlier we said that this number contains three units, two tens and one hundred. But we can also say that this number contains 123 units. Moreover, you can group this number in another way, saying that it contains 12 tens and 3 ones.
Words units, tens, hundreds, replace the multiplicands 1, 10 and 100. For example, in the units place of the number 123 there is a digit 3. Using the multiplicand 1, we can write that this unit is contained in the ones place three times:
100 × 1 = 100
If we add up the results of 3, 20 and 100, we get the number 123
3 + 20 + 100 = 123
The same thing will happen if we say that the number 123 contains 12 tens and 3 ones. In other words, the tens will be grouped 12 times:
10 × 12 = 120
And units three times:
1 × 3 = 3
This can be understood by following example. If there are 123 apples, then you can group the first 120 apples 12 times by 10 pieces:

It turned out to be one hundred and twenty apples. But there are still three apples left. We will call them as "three units"

If we add the results of 120 and 3, we again get the number 123
120 + 3 = 123
You can also group 123 apples into one hundred, two tens and three ones.
Let's group a hundred:

Let's group two dozen:

Let's group three units:

If we add up the results of 100, 20 and 3, we again get the number 123
100 + 20 + 3 = 123
And finally, let's consider the last possible grouping, where the apples will not be distributed into tens and hundreds, but will be collected together. In this case, the number 123 will be read as "one hundred twenty-three units" . This grouping would also be acceptable:

1 × 123 = 123
The number 523 can be read as 3 units, 2 tens and 5 hundreds:
1 × 3 = 3 (three units)
10 × 2 = 20 (two tens)
100 × 5 = 500 (five hundred)
3 + 20 + 500 = 523
You can also read it as 3 ones 52 tens:
1 × 3 = 3 (three units)
10 × 52 = 520 (fifty two tens)
3 + 520 = 523
Another number 523 can be read as 523 units:
1 × 523 = 523 (five hundred twenty-three units)
Where to apply the discharges?
Bits make some calculations much easier. Imagine that you are at the board and solving a problem. You are almost finished with the task, all that remains is to evaluate the last expression and get the answer. The expression to be calculated looks like this:
I don’t have a calculator at hand, but I want to quickly write down the answer and surprise everyone with the speed of my calculations. Everything is simple if you add up the units separately, the tens separately and the hundreds separately. You need to start with the ones digit. First of all, after the equal sign (=) you need to mentally put three dots. These points will be replaced by a new number (our answer):
Now let's start folding. The ones place of the number 632 contains the number 2, and the ones place of the number 264 contains the number 4. This means the ones place of the number 632 contains two ones, and the ones place of the number 264 contains four ones. Add 2 and 4 units and get 6 units. We write the number 6 in the units place of the new number (our answer):
Next we add up the tens. The tens place of 632 contains the number 3, and the tens place of 264 contains the number 6. This means that the tens place of 632 contains three tens, and the tens place of 264 contains six tens. Add 3 and 6 tens and get 9 tens. We write the number 9 in the tens place of the new number (our answer):
And finally, we add up the hundreds separately. The hundreds place of 632 contains the number 6, and the hundreds place of 264 contains the number 2. This means that the hundreds place of 632 contains six hundreds, and the hundreds place of 264 contains two hundred. Add 6 and 2 hundreds to get 8 hundreds. We write the number 8 in the hundreds place of the new number (our answer):
Thus, if you add 264 to the number 632, you get 896. Of course, you will calculate such an expression faster and those around you will begin to be surprised at your abilities. They will think that you are quickly calculating large numbers, but in fact you were calculating small ones. Agree that small numbers are easier to calculate than large ones.
Bit overflow
The digit is characterized by one digit from 0 to 9. But sometimes when calculating numerical expression a bit overflow may occur in the middle of a solution.
For example, when adding the numbers 32 and 14, no overflow occurs. Adding the units of these numbers will give 6 ones in the new number. And adding tens of these numbers will give 4 tens in the new number. The answer will be 46 or six ones and four tens .
But when adding the numbers 29 and 13, an overflow will occur. Adding the ones of these numbers gives 12 ones, and adding the tens gives 3 tens. If you write the resulting 12 units in the units place in the new number, and the resulting 3 tens in the tens place, you will get an error:

The value of the expression 29 + 13 is 42, not 312. What should you do if there is overflow? In our case, the overflow occurred in the units digit of the new number. When we add nine and three units, we get 12 units. And in the units digit you can only write numbers in the range from 0 to 9.
The fact is that 12 units is not easy "twelve units" . Otherwise, this number can be read as "two ones and one ten" . The units digit is for ones only. There is no place for dozens there. This is where our mistake lies. By adding 9 units and 3 units we get 12 units, which can be called in another way two ones and one ten. By writing two ones and one ten in one place, we made a mistake, which ultimately led to an incorrect answer.
To correct the situation, two units need to be written in the ones place of the new number, and the remaining ten must be transferred to the next tens place. After adding the tens in example 29 + 13, we will add to the result the ten that remained when adding the ones.
So, out of 12 units, we write two ones in the ones place of the new number, and move one ten to the next place

As you can see in the figure, we represented 12 units as 1 ten and 2 ones. We wrote two ones in the ones place of the new number. And one ten was transferred to the tens ranks. We will add this ten to the result of adding the tens of the numbers 29 and 13. In order not to forget about it, we wrote it above the tens of the number 29.
Now we add up the tens. Two tens plus one ten is three tens, plus one ten, which remains from the previous addition. As a result, in the tens place we get four tens:
Example 2. Add the numbers 862 and 372 by digits.
We start with the ones digit. In the ones place of the number 862 there is a digit 2, in the ones place of the number 372 there is also a digit 2. This means that the ones place of the number 862 contains two ones, and the ones place of the number 372 also contains two ones. Add 2 units plus 2 units - we get 4 units. We write the number 4 in the units place of the new number:
Next we add up the tens. The tens place of 862 contains the number 6, and the tens place of 372 contains the number 7. This means that the tens place of 862 contains six tens, and the tens place of 372 contains seven tens. Add 6 tens and 7 tens and get 13 tens. A discharge has overflowed. 13 tens is a ten repeated 13 times. And if you repeat the ten 13 times, you get the number 130
10 × 13 = 130
The number 130 is made up of three tens and one hundred. We will write three tens in the tens place of the new number, and send one hundred to the next place:

As you can see in the figure, we represented 13 tens (the number 130) as 1 hundred and 3 tens. We wrote three tens in the tens place of the new number. And one hundred was transferred to the ranks of hundreds. We will add this hundred to the result of adding the hundreds of numbers 862 and 372. In order not to forget about it, we inscribed it above the hundreds of the number 862.
Now we add up the hundreds. Eight hundred plus three hundred is eleven hundred plus one hundred, which is left over from the previous addition. As a result, in the hundreds place we get twelve hundred:
There is also an overflow in the hundreds place here, but this does not result in an error since the solution is complete. If desired, with 12 hundreds you can carry out the same actions as we did with 13 tens.
12 hundred is a hundred repeated 12 times. And if you repeat a hundred 12 times, you get 1200
100 × 12 = 1200
Of the 1200 there are two hundred and one thousand. Two hundred are written into the hundreds place of the new number, and one thousand is moved to the thousand place.
Now let's look at examples of subtraction. First, let's remember what subtraction is. This is an operation that allows you to subtract another from one number. Subtraction consists of three parameters: minuend, subtrahend, and difference. You also need to subtract by digits.
Example 3. Subtract 12 from 65.
We start with the ones digit. The ones place of the number 65 contains the number 5, and the ones place of the number 12 contains the number 2. This means that the ones place of the number 65 contains five ones, and the ones place of the number 12 contains two ones. Subtract two units from five units and get three units. We write the number 3 in the units place of the new number:
Now let's subtract the tens. The tens place of the number 65 contains the number 6, and the tens place of the number 12 contains the number 1. This means that the tens place of the number 65 contains six tens, and the tens place of the number 12 contains one ten. Subtract one ten from six tens, we get five tens. We write the number 5 in the tens place of the new number:
Example 4. Subtract 15 from 32
The ones digit of 32 contains two ones, and the ones digit of 15 contains five ones. You cannot subtract five units from two units, since two units are less than five units.
Let's group 32 apples so that the first group contains three dozen apples, and the second group contains the remaining two units of apples:

So, we need to subtract 15 apples from these 32 apples, that is, subtract five ones and one ten apples. And subtract by rank.
You cannot subtract five units of apples from two units of apples. To perform a subtraction, two units must take some apples from an adjacent group (the tens place). But you can’t take as much as you want, since the dozens are strictly ordered in sets of ten. The tens place can only give two ones a whole ten.
So, we take one ten from the tens place and give it to two ones:

The two units of apples are now joined by one dozen apples. Makes 12 apples. And from twelve you can subtract five, you get seven. We write the number 7 in the units place of the new number:
Now let's subtract the tens. Since the tens place gave one ten to the ones, now it has not three, but two tens. Therefore, we subtract one ten from two tens. There will be only one dozen left. Write the number 1 in the tens place of the new number:
In order not to forget that in some category one ten (or a hundred or a thousand) was taken, it is customary to put a dot above this category.
Example 5. Subtract 286 from 653
The ones digit of 653 contains three ones, and the ones digit of 286 contains six ones. You cannot subtract six ones from three units, so we take one ten from the tens place. We put a dot over the tens place to remember that we took one ten from there:
One ten and three ones taken together make thirteen ones. From thirteen units you can subtract six units to get seven units. We write the number 7 in the units place of the new number:
Now let's subtract the tens. Previously, the tens place of 653 contained five tens, but we took one ten from it, and now the tens place contains four tens. You cannot subtract eight tens from four tens, so we take one hundred from the hundreds place. We put a dot over the hundreds place to remember that we took one hundred from there:
One hundred and four tens taken together make fourteen tens. You can subtract eight tens from fourteen tens to get six tens. We write the number 6 in the tens place of the new number:
Now let's subtract hundreds. Previously, the hundreds place of 653 contained six hundreds, but we took one hundred from it, and now the hundreds place contains five hundred. From five hundred you can subtract two hundred to get three hundred. Write the number 3 in the hundreds place of the new number:
It is much more difficult to subtract from numbers like 100, 200, 300, 1000, 10000. That is, numbers with zeros at the end. To perform a subtraction, each digit has to borrow tens/hundreds/thousands from the next digit. Let's see how this happens.
Example 6
The ones digit of 200 contains zero ones, and the ones digit of 84 contains four ones. You cannot subtract four ones from zero, so we take one ten from the tens place. We put a dot over the tens place to remember that we took one ten from there:
But in the tens place there are no tens that we could take, since there is also a zero there. In order for the tens place to give us one ten, we must take one hundred from the hundreds place for it. We put a dot over the hundreds place to remember that we took one hundred from there for the tens place:
One hundred taken is ten tens. From these ten tens we take one ten and give it to the ones. This one ten taken and the previous zero ones together form ten ones. From ten units you can subtract four units to get six units. We write the number 6 in the units place of the new number:
Now let's subtract the tens. To subtract units, we turned to the tens place after one ten, but at that moment this place was empty. So that the tens place can give us one ten, we take one hundred from the hundreds place. We called this one hundred "ten tens" . We gave one ten to a few. So on this moment The tens place contains not ten, but nine tens. From nine tens you can subtract eight tens to get one ten. Write the number 1 in the tens place of the new number:
Now let's subtract hundreds. For the tens place, we took one hundred from the hundreds place. This means that now the hundreds category contains not two hundred, but one. Since there is no hundreds place in the subtrahend, we move this one hundred to the hundreds place of the new number:
Naturally, perform subtraction like this traditional method quite difficult, especially at first. Having understood the principle of subtraction itself, you can use non-standard methods.
The first way is to reduce a number that has zeroes at the end by one. Next, subtract the subtrahend from the result obtained and add the unit that was originally subtracted from the minuend to the resulting difference. Let's solve the previous example this way:
The number being reduced here is 200. Let's reduce this number by one. If you subtract 1 from 200, you get 199. Now in the example 200 − 84, instead of the number 200, we write the number 199 and solve the example 199 − 84. And solving this example is not particularly difficult. Let's subtract units from units, tens from tens, and simply transfer a hundred to a new number, since there are no hundreds in the number 84:
We received the answer 115. Now to this answer we add one, which we initially subtracted from the number 200
The final answer was 116.
Example 7. Subtract 91899 from 100000
Subtract one from 100000, we get 99999
Now subtract 91899 from 99999
To the result 8100 we add one, which we subtracted from 100000
We received the final answer 8101.
The second way to subtract is to treat the digit in the digit as a number in its own right. Let's solve a few examples this way.
Example 8. Subtract 36 from 75
So, in the units place of the number 75 there is the number 5, and in the units place of the number 36 there is the number 6. You cannot subtract six from five, so we take one unit from the next number, which is in the tens place.
In the tens place there is the number 7. Take one unit from this number and mentally add it to the left of the number 5

And since one unit is taken from the number 7, this number will decrease by one unit and turn into the number 6

Now in the ones place of the number 75 there is the number 15, and in the ones place of the number 36 the number 6. From 15 you can subtract 6, you get 9. We write the number 9 in the ones place of the new number:
Let's move on to the next number, which is in the tens place. Previously, the number 7 was located there, but we took one unit from this number, so now the number 6 is located there. And in the tens place of the number 36 there is the number 3. From 6 you can subtract 3, you get 3. We write the number 3 in the tens place of the new number:
Example 9. Subtract 84 from 200
So, in the ones place of the number 200 there is a zero, and in the ones place of the number 84 there is a four. You cannot subtract four from zero, so we take one unit from the next number in the tens place. But in the tens place there is also a zero. Zero cannot give us one. In this case, we take 20 as the next number.
We take one unit from the number 20 and mentally add it to the left of the zero located in the ones place. And since one unit is taken from the number 20, this number will turn into the number 19

Now the number 10 is in the ones place. Ten minus four equals six. We write the number 6 in the units place of the new number:
Let's move on to the next number, which is in the tens place. Previously, there was a zero there, but this zero, together with the next digit 2, formed the number 20, from which we took one unit. As a result, the number 20 turned into the number 19. It turns out that now the number 9 is located in the tens place of the number 200, and the number 8 is located in the tens place of the number 84. Nine minus eight equals one. We write the number 1 in the tens place of our answer:
Let's move on to the next number, which is in the hundreds place. Previously, the number 2 was located there, but we took this number, together with the number 0, as the number 20, from which we took one unit. As a result, the number 20 turned into the number 19. It turns out that now in the hundreds place of the number 200 there is the number 1, and in the number 84 the hundreds place is empty, so we transfer this unit to the new number:
This method at first seems complicated and meaningless, but in fact it is the easiest. We will mainly use it when adding and subtracting numbers in a column.
Column addition
Column addition is a school operation that many people remember, but it doesn’t hurt to remember it again. Column addition occurs by digits - units are added with units, tens with tens, hundreds with hundreds, thousands with thousands.
Let's look at a few examples.
Example 1. Add 61 and 23.
First, write down the first number, and below it the second number so that the units and tens of the second number are under the units and tens of the first number. We connect all this with an addition sign (+) vertically:
Now we add the units of the first number with the units of the second number, and the tens of the first number with the tens of the second number:
We got 61 + 23 = 84.
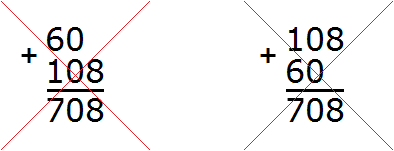
Example 2. Add 108 and 60
Now we add the units of the first number with the units of the second number, the tens of the first number with the tens of the second number, the hundreds of the first number with the hundreds of the second number. But only the first number 108 has a hundred. In this case, the digit 1 from the hundreds place is added to the new number (our answer). As they said at school, “it’s being demolished”:
It can be seen that we have added the number 1 to our answer.
When it comes to addition, it makes no difference in what order you write the numbers. Our example could easily be written like this:
The first entry, where the number 108 was at the top, is more convenient for calculation. A person has the right to choose any entry, but one must remember that units must be written strictly under units, tens under tens, hundreds under hundreds. In other words, the following entries will be incorrect:
If suddenly, when adding the corresponding digits, you get a number that does not fit into the digit of the new number, then you need to write down one digit from the low-order digit and move the remaining one to the next digit.
Speech in in this case This is about the overflow of the bit that we talked about earlier. For example, when you add 26 and 98, you get 124. Let's see how it turned out.
Write the numbers in a column. Units under units, tens under tens:
Add the units of the first number with the units of the second number: 6+8=14. We received the number 14, which does not fit into the units category of our answer. In such cases, we first take out the digit from 14 that is in the ones place and write it in the units place of our answer. In the units place of the number 14 there is the number 4. We write this number in the units place of our answer:
Where to put the number 1 from the number 14? This is where the fun begins. We transfer this unit to the next category. It will be added to the dozens of our answer.
Adding tens with tens. 2 plus 9 equals 11, plus we add the unit that we got from the number 14. By adding our unit to 11, we get the number 12, which we write in the tens place of our answer. Since this is the end of the solution, there is no longer a question of whether the resulting answer will fit into the tens place. We write down 12 in its entirety, forming the final answer.
We received a response of 124.
Using the traditional addition method, adding 6 and 8 units together results in 14 units. 14 units is 4 units and 1 ten. We wrote down four ones in the ones place, and sent one ten to the next place (to the tens place). Then, adding 2 tens and 9 tens, we got 11 tens, plus we added 1 ten, which remained when adding ones. As a result, we got 12 tens. We wrote down these twelve tens in their entirety, forming the final answer 124.
This simple example demonstrates a school situation in which they say “we write four, one in mind” . If you solve examples and after adding the digits you still have a number that you need to keep in mind, write it down above the digit where it will be added later. This will allow you not to forget about it:
Example 2. Add the numbers 784 and 548
Write the numbers in a column. Units under units, tens under tens, hundreds under hundreds:
Add the units of the first number with the units of the second number: 4+8=12. The number 12 does not fit into the units category of our answer, so we take out the number 2 from 12 from the ones category and write it into the units category of our answer. And we move the number 1 to the next digit:
Now we add up the tens. We add 8 and 4 plus the unit that remained from the previous operation (the unit remained from 12, in the figure it is highlighted in blue). Add 8+4+1=13. The number 13 will not fit into the tens place of our answer, so we write the number 3 in the tens place, and move the unit to the next place:
Now we add up the hundreds. We add 7 and 5 plus the unit that remains from the previous operation: 7+5+1=13. Write the number 13 in the hundreds place:
Column subtraction
Example 1. Subtract the number 53 from the number 69.
Let's write the numbers in a column. Units under units, tens under tens. Then we subtract by digits. From the units of the first number, subtract the units of the second number. From the tens of the first number, subtract the tens of the second number:
We received a response of 16.
Example 2. Find the value of the expression 95 − 26
The ones place of the number 95 contains 5 ones, and the ones place of the number 26 contains 6 ones. You cannot subtract six ones from five units, so we take one ten from the tens place. This ten and the existing five ones together make 15 units. From 15 units you can subtract 6 units to get 9 units. We write the number 9 in the units place of our answer:
Now let's subtract the tens. The tens place of 95 used to contain 9 tens, but we took one ten from that place and now it contains 8 tens. And the tens place of the number 26 contains 2 tens. You can subtract two tens from eight tens to get six tens. We write the number 6 in the tens place of our answer:
Let's use it in which each digit included in a number is considered as a separate number. When subtracting large numbers into a column, this method is very convenient.
In the units place of the minuend is the number 5. And in the units place of the subtrahend is the number 6. You cannot subtract a six from a five. Therefore, we take one unit from the number 9. The taken unit is mentally added to the left of the five. And since we took one unit from the number 9, this number will decrease by one unit:

As a result, the five turns into the number 15. Now we can subtract 6 from 15. We get 9. We write the number 9 in the units place of our answer:

Let's move on to the tens category. Previously, the number 9 was located there, but since we took one unit from it, it turned into the number 8. In the tens place of the second number there is the number 2. Eight minus two is six. We write the number 6 in the tens place of our answer:

Example 3. Let's find the value of the expression 2412 − 2317
We write this expression in the column:

In the ones place of the number 2412 there is the number 2, and in the ones place of the number 2317 there is the number 7. You cannot subtract seven from two, so we take one from the next number 1. We mentally add the taken one to the left of the two:

As a result, two turns into the number 12. Now we can subtract 7 from 12. We get 5. We write the number 5 in the units place of our answer:

Let's move on to tens. In the tens place of the number 2412 there used to be the number 1, but since we took one unit from it, it turned into 0. And in the tens place of the number 2317 there is the number 1. You cannot subtract one from zero. Therefore, we take one unit from the next number 4. We mentally add the taken unit to the left of zero. And since we took one unit from the number 4, this number will decrease by one unit:

As a result, zero turns into the number 10. Now you can subtract 1 from 10. You get 9. We write the number 9 in the tens place of our answer:

In the hundreds place of the number 2412 there used to be a number 4, but now there is a number 3. In the hundreds place of the number 2317 there is also a number 3. Three minus three equals zero. The same goes for the thousand places in both numbers. Two minus two equals zero. And if the difference between the most significant digits is zero, then this zero is not written down. Therefore, the final answer will be the number 95.
Example 4. Find the value of the expression 600 − 8
In the units place of the number 600 there is a zero, and in the units place of the number 8 this number itself is located. You can’t subtract eight from zero, so we take one from the next number. But next number this is also zero. Then we take the number 60 as the next number. We take one unit from this number and mentally add it to the left of zero. And since we took one unit from the number 60, this number will decrease by one unit:

Now the number 10 is in the ones place. From 10 you can subtract 8, you get 2. Write the number 2 in the units place of the new number:
Let's move on to the next number, which is in the tens place. There used to be a zero in the tens place, but now there is a number 9 there, and in the second number there is no tens place. Therefore, the number 9 is transferred to the new number:
Let's move on to the next number, which is in the hundreds place. There used to be a number 6 in the hundreds place, but now there is a number 5 there, and in the second number there is no hundreds place. Therefore, the number 5 is transferred to the new number:
Example 5. Find the value of the expression 10000 − 999
Let's write this expression in a column:

In the units place of the number 10000 there is a 0, and in the units place of the number 999 there is a number 9. You cannot subtract nine from zero, so we take one unit from the next number, which is in the tens place. But the next digit is also zero. Then we take 1000 as the next number and take one from this number:

The next number in this case was 1000. Taking one from it, we turned it into the number 999. And we added the taken unit to the left of zero.
Further calculations were not difficult. Ten minus nine equals one. Subtracting the numbers in the tens place of both numbers gave zero. Subtracting the numbers in the hundreds place of both numbers also gave zero. And the nine from the thousands place was moved to a new number:

Example 6. Find the value of the expression 12301 − 9046
Let's write this expression in a column:

In the units place of the number 12301 there is the number 1, and in the units place of the number 9046 there is the number 6. You cannot subtract six from one, so we take one unit from the next number, which is in the tens place. But in the next digit there is a zero. Zero can't give us anything. Then we take 1230 as the next number and take one from this number:

Numerals – independent part speech, indicating the number of objects, their serial number, as well as total. Depending on their purpose, numerals are divided into three broad groups, each of which is described in detail in this article. It also provides grammatical signs of numerals and visual examples.
Numerals are studied in 6th grade. This part of speech can denote the number of some objects, the number of an object in order, as well as the quantity as a whole. Depending on this value they are divided into 3 large groups.
Numerals can be quantitative, ordinal And collective. Each of these groups of words has its own characteristics.
Table“Lexico-grammatical categories of numerals in the Russian language” with examples
TOP 5 articleswho are reading along with this
For example: ten, thirty, seven hundred eleven.
Numerals of this category can have different case forms. For some cardinal numerals, the form of gender and number is determined. The following table reflects all the features of words in this category.
Changing cardinal numbers
Numeral |
How it changes | Examples |
| 1 | By gender, number and case | One - one, alone, alone |
| 2,3, 4 | By cases and genders | Two, two, two, three |
| 5-20,30 | By cases, as a being of the 3rd class. | Wed: tablecloth - ten, eleven |
| 50-80, 200-900 | By case, both parts change | Fifty, three hundred |
| 40, 90, 100 | According to cases, they have only 2 forms | Forty - forty
One hundred - one hundred Ninety - ninety |
| 1000 | By cases as a noun. 1st class | Wed: Candles - a thousand |
| 1000000, | By cases as a noun. 2nd class | Wed: home - million - billion |
Ordinal numbers: grammatical features
Numerals of this category are grammatically similar to adjectives. They can also have forms of different cases, genders and numbers.
Ordinal numbers can also have different structures. When declension of compound numerals of this category changes only the last word. For example.
Depending on the gas pressure, electrode configurations and external circuit parameters, there are four types of independent discharges:
- glow discharge;
- spark discharge;
- arc discharge;
- corona discharge.
1. Glow discharge occurs when low pressures. It can be observed in a glass tube with flat metal electrodes soldered at the ends (Fig. 8.5). Near the cathode there is a thin luminous layer called cathode luminous film 2.
Between the cathode and the film there is Aston's dark space 1. To the right of the luminous film is placed a weakly luminous layer called cathode dark space 3. This layer goes into a luminous area, which is called smoldering glow 4, the smoldering space is bordered by a dark gap - Faraday dark space 5. All of the above layers form cathode part glow discharge. The rest of the tube is filled with glowing gas. This part is called positive column 6.
As the pressure decreases, the cathode part of the discharge and the Faraday dark space increase, and the positive column shortens.
Measurements showed that almost all potential drops occur in the first three sections of the discharge (Aston's dark space, cathode luminous film and cathode dark spot). This portion of the voltage applied to the tube is called cathode potential drop.
In the region of the smoldering glow, the potential does not change - here the field strength is zero. Finally, in the Faraday dark space and positive column the potential slowly increases.
This potential distribution is caused by the formation of a positive space charge in the cathode dark space, due to the increased concentration of positive ions.
Positive ions, accelerated by the cathode potential drop, bombard the cathode and knock electrons out of it. In the Aston dark space, these electrons, flying without collisions into the region of the cathode dark space, have high energy, as a result of which they more often ionize molecules than excite them. Those. The intensity of the gas glow decreases, but many electrons and positive ions are formed. The resulting ions initially have a very low speed and therefore a positive space charge is created in the cathode dark space, which leads to a redistribution of potential along the tube and the occurrence of a cathode potential drop.
Electrons generated in the cathode dark space penetrate into the region of smoldering glow, which is characterized by a high concentration of electrons and positive ions and a polar space charge close to zero (plasma). Therefore, the field strength here is very low. In the region of the smoldering glow, an intense recombination process takes place, accompanied by the emission of energy released during this process. Thus, the smoldering glow is mainly a recombination glow.
From the region of smoldering glow into Faraday dark space, electrons and ions penetrate due to diffusion. The probability of recombination here drops greatly, because the concentration of charged particles is low. Therefore, there is a field in Faraday dark space. The electrons entrained by this field accumulate energy and often eventually create the conditions necessary for the existence of a plasma. The positive column represents gas-discharge plasma. It acts as a conductor connecting the anode to the cathode parts of the discharge. The glow of the positive column is caused mainly by transitions of excited molecules to the ground state.
2. Spark discharge occurs in gas usually at pressures on the order of atmospheric pressure. It is characterized by an intermittent form. By appearance a spark discharge is a bunch of bright zigzag branching thin stripes that instantly penetrate the discharge gap, quickly extinguish and constantly replace each other (Fig. 8.6). These strips are called spark channels.
 |
T gas = 10,000 K ~ 40 cm I= 100 kA t= 10 –4 s l~ 10 km |
After the discharge gap is “broken” by the spark channel, its resistance becomes small, and a short-term current pulse passes through the channel great strength, during which only a small voltage falls on the discharge interval. If the source power is not very high, then after this current pulse the discharge stops. The voltage between the electrodes begins to increase to its previous value, and the gas breakdown is repeated with the formation of a new spark channel.
In natural natural conditions the spark discharge is observed in the form of lightning. Figure 8.7 shows an example spark discharge– lightning, duration 0.2 ÷ 0.3 with a current strength of 10 4 – 10 5 A, length 20 km (Fig. 8.7).


3. Arc discharge . If after receiving a spark discharge from powerful source gradually reduce the distance between the electrodes, then the discharge from intermittent becomes continuous, and new form gas discharge, called arc discharge(Fig. 8.8).
 |  |
|
| ~ 10 3 A | ||
| Rice. 8.8 | ||
In this case, the current increases sharply, reaching tens and hundreds of amperes, and the voltage across the discharge gap drops to several tens of volts. According to V.F. Litkevich (1872 - 1951), the arc discharge is maintained mainly due to thermionic emission from the cathode surface. In practice, this means welding, powerful arc furnaces.
4. Corona discharge (Fig. 8.9).occurs in a strong inhomogeneous electric field with relatively high pressures gas (about atmospheric). Such a field can be obtained between two electrodes, the surface of one of which has a large curvature (thin wire, tip).
 |
 |
The presence of a second electrode is not necessary, but its role can be played by the nearest, surrounding grounded metal objects. When electric field near an electrode with a large curvature reaches approximately 3∙10 6 V/m, a glow appears around it, looking like a shell or crown, which is where the name of the charge comes from.